
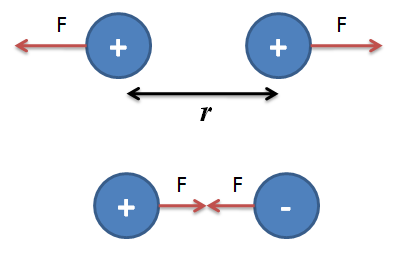
People have noticed, I guess what you couldĬall electrostatics, for a large swathe of But the question is, what causes, how can we predict how strong the force of attraction or repulsion is going to be between charged particles? And this was a question So these things are going to, these two things are going How these different charges, this framework that we've created, how these things start to This charge is a property of matter that we've started to observe. So if I have a positiveĪnd I have a negative they are going to attract each other.

But if they have different charges, they are going to attract each other. So in either of these cases these things are going Things have the same charge, so they're either both positive, or they are both negative, then they are going to repel each other. So we've already started to familiarize ourselves So a beam of gluons would not just pass through another beam of gluons like one laser beam does pass through another. It also means that the particles which are exchanged to produce the strong force, gluons, carry "color charge" themselves, unlike photons which have no electric charge. The "charge" for the strong force is called "color charge", and comes in THREE types, which physicists call red, green, and blue. This means that the force-carrying particle of the strong force (called "gluons") DOES come in more than two types. Other forces have other symmetries, though! For example, the symmetry of the strong force (which holds the quarks together inside protons and neutrons, and holds the protons and neutrons together inside atomic nuclei) is a much more exotic symmetry called "SU(3)". This shows that the symmetry of electromagnetism is U(1), and thus that electric charge comes in integer chunks. Charged particles (electrons and protons) affect (produce, absorb, bend) light, and light (or radio waves or x-rays, they are all photons) affects charged particles, but light passes straight through other light.
It turns out that if the symmetry group is not U(1), then the force-carriers must themselves carry some kind of charge, and that would mean that photons would significantly affect other photons! But if you do the experiment of crossing two laser beams, you can see that light (photon beams) has no direct effect on other light.
Now, you might then ask, "But how do we know that the symmetry is U(1)?" The answer to that would be that we can only guess what the symmetry is and then do experiments to find out. the size of that chunk can only be discovered by experiment). So it's not exactly that there are "two types" of electric charge, but more like "electric charge must come in chunks of. Putting this together with a lot of very advanced math, the result is that electric charge has to come in integer amounts. If you think about a circle with some points on it labeled, the only thing you can do to it that will leave it exactly the same is rotate it an integer number of times. Every force also has a mathematical symmetry associated with it, and for the electric force that symmetry is the symmetry of the circle (this is called the "U(1) symmetry group"). For the electric force, the force-carrier is the photon, which is sort of like a "chunk" of oscillating electromagnetic field which flies around at the speed of light. So, for every force, it turns out that there is not really a "field" in the way we learn in intro physics, but instead the "force" is caused by the exchange of some particle.
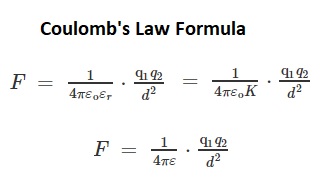
For hundreds of years, people thought Newton and Coulomb had found the EXACT FINAL PERFECT TRUE laws of physics, and only in the 20th century, when relativity and quantum mechanics were discovered, did physicists learn that the truth is totally, radically different from these laws for very tiny objects and high speeds. Newton's law of gravity, Coulomb's law of electrostatics, and Maxwell's laws of electromagnetism are all just approximately true, for systems which are on the human scale of time, space, energy, and speed. First, you have to know this secret: almost everything you learn in the first three years of physics is not really true. Great question! A complete answer to this requires very advanced mathematics, unfortunately, but I will try to give a taste of the idea.


 0 kommentar(er)
0 kommentar(er)
